Jan 22, 2024

This morning, after breakfast in the cafeteria, students came to the first lecture of the week, featuring presenter Nick Rauh from the Seattle Universal Math Museum on “tensegrity polyhedra.” (“Tensegrity” was apparently coined by the famous architect Buckminster Fuller.) Using small dowels and rubber bands, students were challenged to create “tensegrity units” consisting of two dowels joined at each end by rubber bands, with an additional rubber band running the length of the dowels pinched between the two dowels at each end. These units could be combined to form larger constructions. Nick led the students through one such larger construction. Here is Adam’s attempt at creating the cuboctahedron:


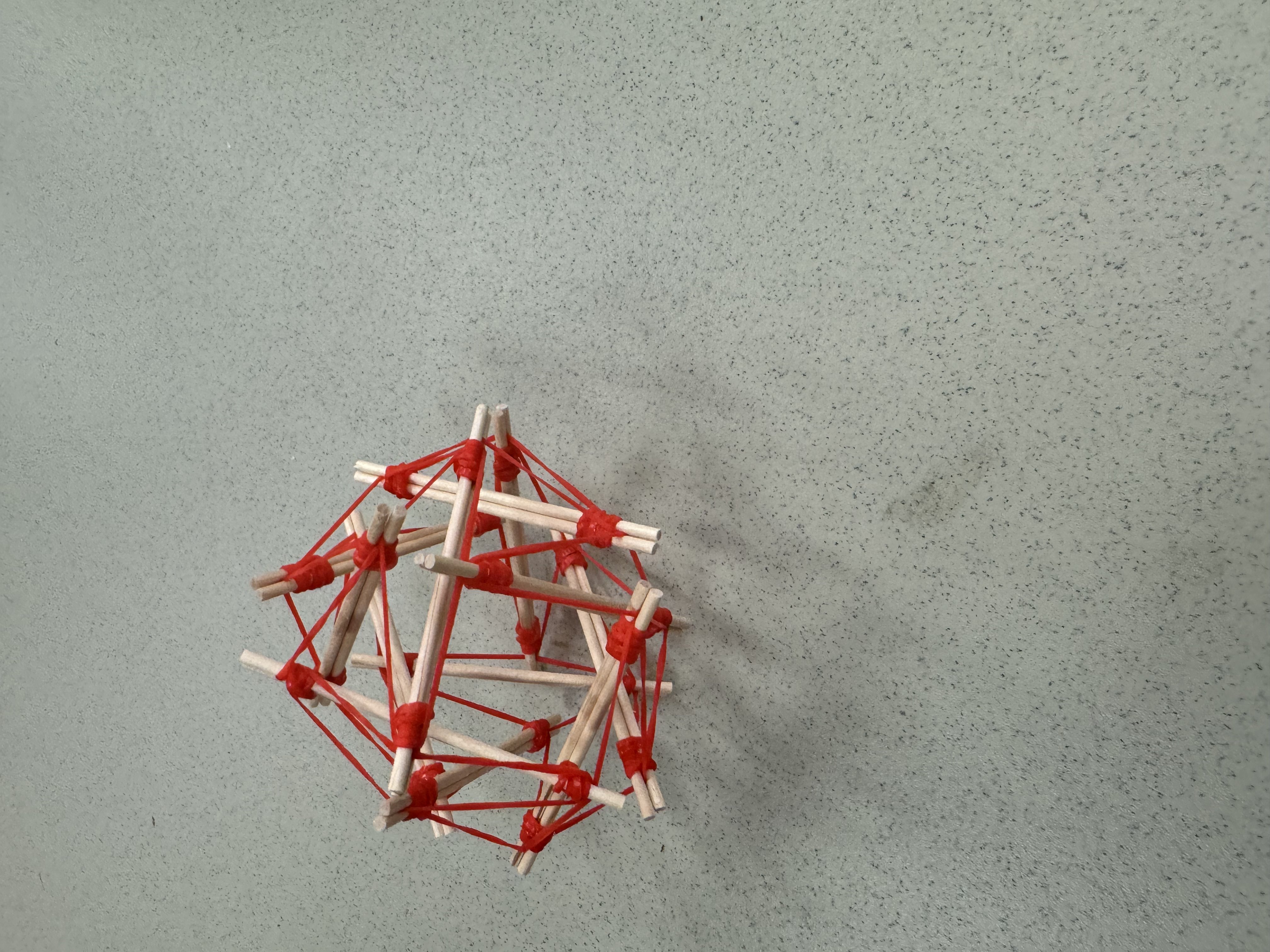
Here is another of the constructions that can be made from directions at the website listed below (depending on how you look at it, either an octahedron or an icosahedron):


Nick explained how these constructions are related to the five Platonic solids, solids with every side made up of the same regular shape, and other polyhedra that are not Platonic because they use more than one shape (for example, triangles AND squares), but are still the same at every corner. Some of them, such as the square antiprism, are quite complicated! Nick says you can find information on the materials he used for this lecture, more information on how the constructions represent the solids, and directions to be able to build a few other possible constructions at https://maththem.blogspot.com/2024/02/tensegrity-polyhedra.html?m=1

Next up, Professor Victor Barranca of Swarthmore College spoke to our students about applied mathematics, his field of interest. He began by asking our students, “What Is Mathematics?” This may seem like a simple question to ask, but the answer is a bit more complicated than you might think. Mathematics is not just numbers or even patterns of numbers. It’s also about geometric shapes and their patterns. One possible definition of mathematics proposed by Professor Barranca: a system for the application of abstract reasoning to real-world situations.

Professor Barranca went on to discuss the development of mathematics from early civilization to today, from counting, to arithmetic, to measuring/building, to Fibonacci, and eventually to the highly sophisticated mathematics we now have. Along the way students were asked to think about what makes humans uniquely suited to mathematics. Some animals have a sense of numbers; why are we so much better at math? Is it just our language ability? Or the percentage of our energy consumption devoted to our unusually large brains?

Students explored real-world applications of math, such as uses in financial markets, where to place armor on aircraft to provide the most protection in war, maximizing the efficiency of medical scans, modeling the spread of disease, weather prediction, modern data storage, computer search engines, computer graphics and animation, and even the functioning of the human brain. Dr. Barranca ended his talk by discussing the “birthday match” problem (what is the probability that two students in a class of 23 will have the same birthday?) and the Monty Hall Problem, a very well-known problem based on the game show Let’s Make a Deal. In this show, there are three doors. Behind two of these doors is a goat and behind the third door is a car. A contestant chooses one of the doors, but before the door is opened one of the doors that was NOT chosen is opened and reveals a goat. The contestant is then offered an opportunity: stick with the door they originally chose, or switch to the remaining unopened door. What would you do? If you want to learn more about this problem, you can do so at Monty Hall Problem.

The students had some time for lunch in the cafeteria and socializing before returning for more presentations. Our first lecturer after lunch today might have looked familiar to our students, because we were lucky enough to have a second lecture from this morning’s first lecturer, Nick Rauh!

This afternoon Nick taught the student’s “Grundy’s Game.” The students paired off for the game and started with a pile of 8 chips between them. Players took turns splitting any pile they liked into two piles, but the two piles had to be of unequal size. Whichever player was the last to be able to split a pile was the winner. Nick challenged the students to try to come up with the optimal strategy for the game. Questions raised included: What's a strategy for winning? Does it matter who makes the first move? What happens if we start with 9 chips? 10 chips?

Once the students came up with the optimal strategies for both 8-chip and 9-chip variations of the game, the group discussed what general rules could be derived. For instance, piles of either 1 or 2 chips cannot be divided, so getting down to piles of that size is the key to the game. Extrapolating up from there, students were able to see how a pile of 7 would always mean the end of the game for the player who had to make the next move. Eventually, this analysis leads to a table of “Grundy Values,” which are numbers of chips that guarantee that the first/next player to move is the loser if both players use good strategy. The students’ final challenge was to try to invent a strategy for a game starting with 32 chips in the pile!

Our final presentation of the day was from Keith Calkins, a veteran math educator and physics PhD who has helped Math League with editing questions and has presented to our students many times in the past. The presentation started with each student choosing a favorite number and then discussing the properties of the chosen numbers. (Counting numbers? Whole? Rational? Real?) The group explored different ways to think about and even to express numbers, for example in Roman numerals or in base 2. Bases in particular were discussed, including base e (an irrational base) and base -3 (a negative base)!

After the dinner break, students were treated to a movie night, complete with snacks and a film shown on a big screen. Tonight’s movie was the classic, MOVIE.